|
Perhaps the most
obvious macro level parameter is the number of each type of entity of
which the system is composed. Nk will represent the of
number of entities of type k in the system under consideration.
The second macroscopic
parameter I wish to consider is the total internal relation energy of
the system. This is the sum of all the relation kinetic and relation
potential energy of the system as defined above under relationship
dynamics. I shall call it E.
The third macroscopic
parameter I wish to consider is the relation interdependence
configuration space volume. I define it as
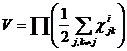 . .
For simplicity I shall
call this macroscopic parameter relation volume. Conceptually it can be
thought of as representing the size of the interdependence of the entire
system. The larger this volume the greater the average distance between
entities and therefore the smaller the interdependence and, of course,
vice versa.
Introduced this way
this parameter and its definition may seem to be somewhat mysterious
with little justification. Indeed, I could present some qualitative
reasons indicating its significance; however its value will ultimately
be determined by its usefulness in a quantitative formulation.
Therefore, I will let its merit rest solely upon how it fits into that
quantitative formulation.
The macroscopic
parameters E, V, Nk have a common property that will prove to
be very significant. Consider two systems characterized by EA,
VA, NkA and EB, VB,
NkB. If system A and system B are now combined,
then
EC
= EA + EB
VC
= VA + VB
NkC
= NkA + NkB
Parameters that have values in a composite system equal to the sum of
the values in each of the subsystems are called extensive
parameters. Extensive parameters play a key role throughout
thermodynamics theory.
Callen [1960, 9]
I shall find occasion
to use various ratios of the extensive parameters. In a system
containing r entity types I shall call the r ratios
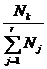 entity
fractions, and the quantity entity
fractions, and the quantity
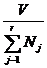 the
average entity volume. the
average entity volume.
ç
Prior Page of Text
Next Page of Text
è
(C) 2005-2014 Wayne M. Angel.
All rights reserved. |
